Canan Özeren, Dokuz Eylül University. Date: 6th of December, 2023, Wednesday, Time: 13.30 – 14.30. Place: Dokuz Eylül Univ., Tınaztepe Campus, Faculty of Science, Department of Mathematics, Room B206 (Online-Sakai-Graduate Meetings).
Abstract: Torsion-free covers exist for abelian groups (see [1]). The coGalois group of automorphisms a torsion-free cover 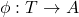 of an abelian group
of an abelian group  is defined in [2] as the group of
is defined in [2] as the group of 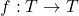 s.t.
s.t. 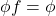 and is denoted by
and is denoted by 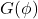 . The abelian groups for which the coGalois group is trivial were characterized in [3]. The notion of coGalois group can be defined in any category where we have a covering class. In [4], coGalois groups have been studied in the category of representations of the quiver
. The abelian groups for which the coGalois group is trivial were characterized in [3]. The notion of coGalois group can be defined in any category where we have a covering class. In [4], coGalois groups have been studied in the category of representations of the quiver  . We talk about the existence and uniqueness of the torsion free-cover of an object in
. We talk about the existence and uniqueness of the torsion free-cover of an object in  (see [5]).
(see [5]).
References:
[1] E. Enochs: Torsion-free covering modules. (1963).
[2] E. Enochs, J. R. Rozas and L. Oyonarte: Compact coGalois groups. (2000).
[3] E. Enochs and J. Rada: Abelian groups which have trivial absolute \\ coGalois group. (2005).
[4] Paul Hill, Abelian group pairs having a trivial coGalois Group. (2006).
[5] Molly Dunkum Wesley, Phd Thesis at The Graduate School University of Kentucky. (2005)
![]() . Next, we will introduce group codes and examine the relationship between cyclic group codes and cyclic codes.
. Next, we will introduce group codes and examine the relationship between cyclic group codes and cyclic codes. 